Lecture 19: Minimum Spanning Trees, Part 2

COSC 311 Algorithms, Fall 2022

$ \def\compare{ {\mathrm{compare}} } \def\swap{ {\mathrm{swap}} } \def\sort{ {\mathrm{sort}} } \def\insert{ {\mathrm{insert}} } \def\true{ {\mathrm{true}} } \def\false{ {\mathrm{false}} } \def\BubbleSort{ {\mathrm{BubbleSort}} } \def\SelectionSort{ {\mathrm{SelectionSort}} } \def\Merge{ {\mathrm{Merge}} } \def\MergeSort{ {\mathrm{MergeSort}} } \def\QuickSort{ {\mathrm{QuickSort}} } \def\Split{ {\mathrm{Split}} } \def\Multiply{ {\mathrm{Multiply}} } \def\Add{ {\mathrm{Add}} } \def\cur{ {\mathrm{cur}} } \def\gets{ {\leftarrow} } $

Overview

1. Midterm Comments
2. Prim’s Algorithm, Again
3. Kruskal’s Algorithm

Overall Midterm Comments

1. Interpretation of grades
2. Very happy with grade distribution
3. Two more consistent issues
   • Question 2 ($0$, $\Theta$, $\Omega$)
   • Question 3 (D&C Algorithm)

Question 3 Illustration

MSTs

Input:

• a weighted graph $G = (V, E)$ with edge weights $w$

Output:

• a set $F$ of edges in $E$ such that

  1. $(V, F)$ is connected
  2. sum of weights of edges in $F$ is minimal among all connected sub-graphs of $G$

The graph $T = (V, F)$ is called a minimum spanning tree of $G$

Prim’s Algorithm

  PrimMST(V, E):
    initialize set S = {v} with v arbitrary
    initialize set F = {} of MST edges, priority queue Q
    for each neighbor x of v
      add (v, x) to Q with priority w(v, x)
    while Q is not empty
      (u, v) <- removeMin(Q)
      if S doesn't contain v
        add (u, v) to F
        for each neighbor x of v
          add (v, x) to Q with priority w(v, x)
    return (S, F)

Prim Illustration

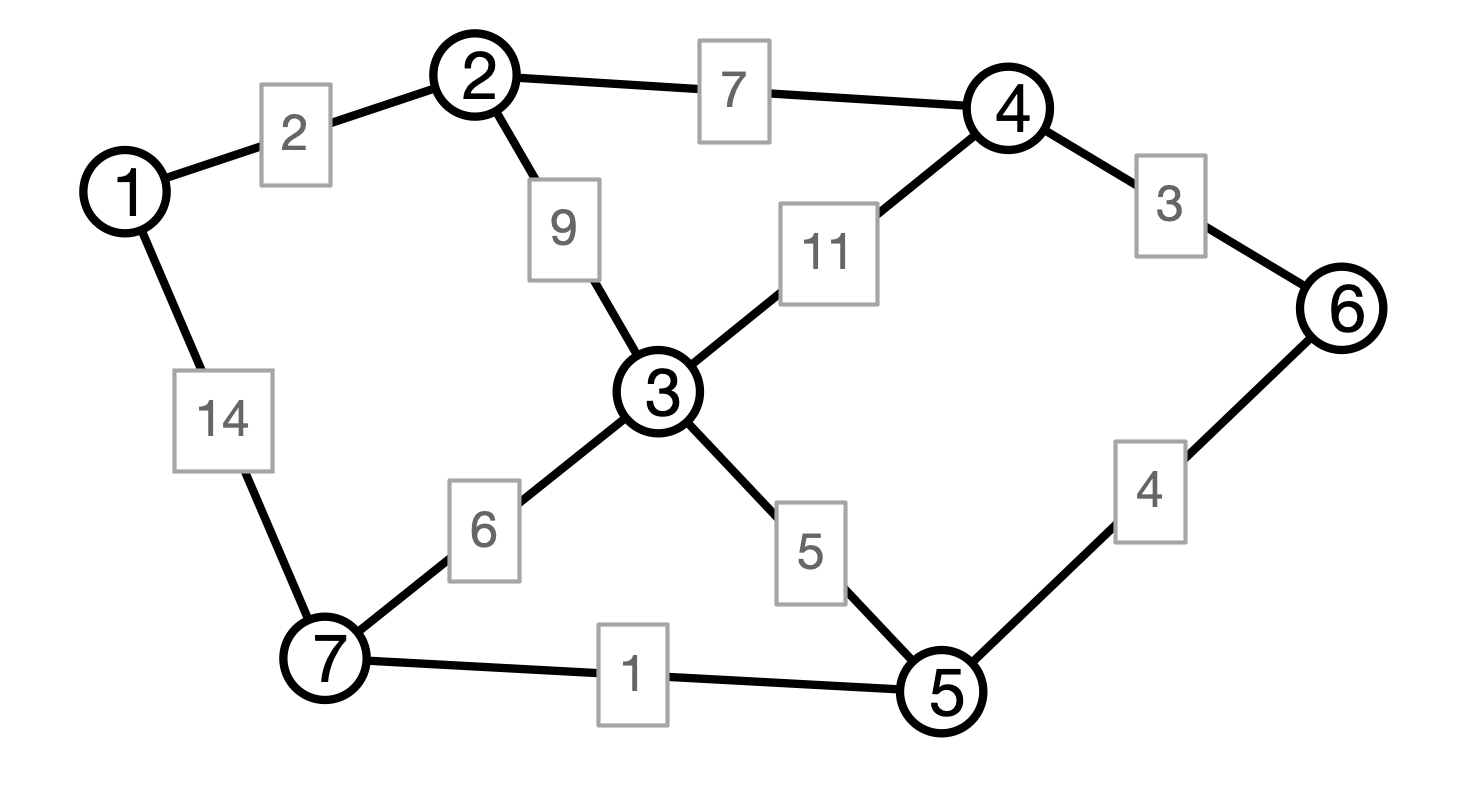
Cuts in Graphs

Definition. Let $G = (V, E)$ be a graph. A cut in $G$ is a partition of $V$ into two (non-empty) subsets $U$ and $V - U$.

Cuts and MSTs

Cut Claim. Suppose:

• $G = (V, E)$ is a weighted graph (with distinct edge weights)
• $U, V - U$ a cut in $G$
• $T = (V, F)$ an MST
• $e = (u, v)$ is the minimum weight edge that crosses the cut
  • $u \in U$ and $v \in V - U$

Then:

• $T$ contains the edge $e$

Cut Claim Illustration

Prim, Again

  PrimMST(V, E):
    initialize set S = {v} with v arbitrary
    initialize set F = {} of MST edges, priority queue Q
    for each neighbor x of v
      add (v, x) to Q with priority w(v, x)
    while Q is not empty
      (u, v) <- removeMin(Q)
      if S doesn't contain v
        add (u, v) to F
        for each neighbor x of v
          add (v, x) to Q with priority w(v, x)
    return (S, F)

Prim Correctness I

Cut claim $\implies$ Prim produces an MST.

Why?

Prim Correctness II

First Conclusion. Every edge $e$ added by Prim’s algorithm is in the MST.

Still to show. All MST edges are added.

Why is this so?

Prim Running Time?

  PrimMST(V, E):
    initialize set S = {v} with v arbitrary
    initialize set F = {} of MST edges, priority queue Q
    for each neighbor x of v
      add (v, x) to Q with priority w(v, x)
    while Q is not empty
      (u, v) <- removeMin(Q)
      if S doesn't contain v
        add (u, v) to F
        for each neighbor x of v
          add (v, x) to Q with priority w(v, x)
    return (S, F)

Conclusion

Prim’s algorithm:

• computes an MST in $G$
• if efficient priority queue is used, running time is $O(m \log n)$

Prim’s algorithm is greedy

• to grow a tree, always add the lightest outgoing edge

MSTs, Another Way

Prim:

• Grow tree greedily from a single seed vertex
• Maintain a (connected) tree

Edge Centric View:

• Maintain a collection of edges (not necessarily a tree)
• Add edges to collection to eventually build an MST

Questions:

• How to prioritize edges?
• How to determine whether or not to include an edge?

Picture

Kruskal’s Algorithm

  Kruskal(V, E, w):
    C <- collection of components
      initially, each vertex is own component
    F <- empty collection
    # iterate in order of increasing weight
    for each edge e = (u, v) in E 
      if u and v are in different components then
        add (u, v) to F
        merge components containing u and v
      endif
    endfor
    return F

Kruskal Illustration

Kruskal Correctness I

Claim 1. Every edge added by Kruskal must be in every MST.

Why?

Kruskal Correctness II

Claim 2. Kruskal produces a spanning tree.

Why?

Conclusion

Theorem. Kruskal’s algorithm produces an MST.

Question. How could we implement Kruskal’s algorithm efficiently? What is its running time?

Next Time

1. Efficiency of Kruskal
2. Interval Scheduling